HOME

PRESUMED IMPOSSIBILITIES, continued1, 2

PHOTOGRAPHY, continued1, 2, 3, 4

PORTRAITURE, continued1, 2, 3

COMMERCIAL ART, continued1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12, 13, 14, 15, 16, 17, 18, 19, 20, 21, 22, 23, 24, 25

INVENTION

AUTOBIOGRAPHY, continued1, 2, 3, 4

So I was anxious to see it, and I did a drawing of it now despite my difficulties in making a livelihood in the city. Although I have reservations about the "wedding-cake" architecture, the building is to me, in its soaring rise to the skies, a monument to American know-how, not destroyed by terrorists with the destruction of any of its results.

The next illustration (I try to enliven the pages with pictures whenever suitable) I include for several reasons. It is work I did at a mentioned studio for their own intended publicity, before I went to a hospital again, in Denver, for a diagnosed minimal case of tuberculosis, as I also note on that page.

One reason for the picture here is that it may make sense in this autobiography to show people involved in my life, including bosses. The likeness of the tall handsome guy at left is, somewhat altered, of my boss at that studio. The painting, in the original at least twice the size, is after a black & white "8 by 10 glossy", the kind of photo used by illustrators. In this one my boss, Bill Keyes, posed with a professional model. He was really fair in color and maybe had less hair. The girl seems an exceptional beauty.

The picture was for some reason not used, but among my ideas was possibly doing illustrations for magazines, done a lot then for such as The Saturday Evening Post or Ladies Home Journal. That was just a pipe dream. I wasn't settled enough for all the prerequisites demanded. Anyway, painting this picture was easy for me after the movie posters in Prague, and I aimed at qualities like flat color areas, as in the suit and its shadows, contrasted with more modeled forms as in the faces. To explain more of my work, beside the special sections on this website, is then also a reason for this inclusion.

With regard to my going then to the National Jewish Hospital in Denver, I showed elsewhere some graphic things I occupied myself with there, and also photographs I took of fellow patients in other settings. This time I might add a pair of photographs taken (not with my camera) on the roofs of the hospital.

As I also indicated elsewhere, after my hospital stay, which I somehow only vaguely remember to have lasted about a year, I was offered a job through an acquaintance I met, who was a friend of my future boss to whom he recommended me. I did have some work to show, which helped in getting the job. My above previous boss, Bill Keyes, was, in anticipation of my returning to the New York job instead, kind enough to store, while I was hospitalized, the only car I have ever owned, a '51 Ford. But I preferred the job in Denver, because I was offered a good constant salary and a comfortable place to work, without the hectic unpredictability of the other job.

Accordingly on returning to New York and getting my car out of storage, I drove it to Denver. On the way I got to see America better, especially by driving through the vast open plains, giving a pioneering feeling. Playing the car-radio during the long ride helped to contribute to the mood. Below I am depicting the adventure, with what really was a navy-blue car but is shown a bit lighter because my computer equipment has trouble with the color blue.

As I mentioned on another page that pictures autos, I consider today's car-designs mostly ugly, particularly their imitative v-shaped grills and headlights (industrial design belongs to an art form, always part of my interests). I felt previously that on my Ford the two shapes in front resembling airplane engines made little sense, but on the whole I find the balanced design far superior to what is done today.

Depicted next are my employers in Denver, partners in the company they named Hoflund-Schmidt Typographic Service. The first shown person was my actual boss, Ray Schmidt, who handled the art department. The second is Mr. Hoflund, the typography head. He really was more solemn, and I had problems with him more than with Ray. Layout men, ad designers, of which I was one, customarily specify the typefaces, point sizes, and the rest of what they design, and Mr. Hoflund often decided on his own on these, which of course bothered me.

These two pictures, displaying the halftone dots used in printing, are corresponding magnifications taken from an ad by the company. People often cut off the necks for these designs, as done here by someone, adding a few cartoon lines for the torsos.

Much of what I did at this job, which lasted about six years, is shown on the "commercial art" pages of this website. In the "photography" pages I also show some pictures taken on a trip to Aspen during this time. I went there, with the below companions, to attend a design conference sponsored by Westvaco, the West Virginia Paper Company, known for its advertisements and support of good design. Below are two more pictures from that trip.

Included below is some more from my life in Denver.

When the relationship didn't work out, I was very despondent and not long after, in 1958, drove back to New York. Years later my emotional states became stable, and I came to favor living alone, which enabled me to be creative in ways I couldn't have been otherwise.

5 October 2005

The following pictures I am adding a bit hesitantly, because my old ponim is nothing to brag about. I am jumping here from the preceding pictures more than half a century, the present photos taken last Saturday, and a reason I am using them here is to show that one can be reasonably muscular at age 80. I am not saying this for vanity reasons, but to somehow demonstrate along with the other contents of this website that many things can be accomplished that are thought of otherwise. Likewise it is thought that intellectual creativity is not a characteristic of old age, and my efforts are to convince that this is not so, my having begun both physical and mental development of greater degree after 40.

This addition to my autobiography may be the last one, since it seems to me at this time that apart from my activities in different areas I deal with separately on this website, my life has undergone no significant changes after moving back to New York. Roughly all I did was hold a job for a few years, and moved from Queens to Manhattan about forty years ago, where I have lived in the same apartment since.

31 May 2006

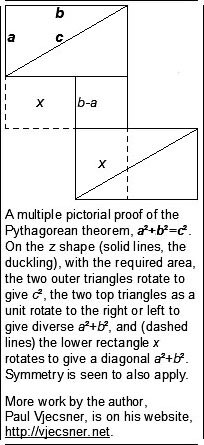
Below I am after all adding another item, representing some of my activities after leaving my last job. As can be gathered from other parts of this website, among the intellectual interests I turned to was mathematics, often concerning geometry, as seen under PRESUMED IMPOSSIBILITIES or INVENTION. (Recently I also ran four ads on the subject in Scientific American of November 2005, p.111, December 2005, p.31, March 2006, p.101, and April 2006, p.24. They can be found at public libraries. [The ads are shown now below.]) (Let me not forget to note again: a lot more, beside other subjects, is in my book.)

The below, although showing some graphics, does not belong to geometry but to so-called number theory, more specifically prime numbers, which are those whole numbers after 1 that are not divisible by any other whole number beside 1. About 30 years ago I managed to get one article a piece on prime numbers accepted by the New York State Mathematics Teacher's Journal (May 1976) and The New Jersey Mathematics Teacher (Winter 1977), the last of which contained a form of what I am now showing.

One of the questions since antiquity has been how prime numbers can be generated, how they can up to a given number be located. As answer was given a method known as the sieve of Eratosthenes, after the ancient Greek thinker and which is continued to be taught to date (note a recent other item by Eratosthenes I worked on). What he did rather understandably was list all whole numbers after 1 up to say 100, then successively mark out all multiples of 2, of 3, etc., with the remaining numbers of course the primes. The procedure is obviously lengthy, by having to list all the numbers dealt with, and when the prime numbers get high, it can be a burden to do addition for each of their succeeding multiple.

In place of that sieve I devised another, which reduces the labor drastically. Its underlying basis is that after 2 and 3 all prime numbers flank the number 6 or its multiples, because all other numbers outside the flanked ones, as well as the flanked ones, are multiples of 2 or 3. Accordingly it is only necessary to list 6 and its multiples (underlined in the illustration here) up to say again 100. After, by mainly a simple counting process, the flanks that are not prime, those that are multiples, are marked out (black boxes), with the remaining flanks the primes (shown in the image, in bold type, for survey).

The process employed is the following (proof of its correctness will be given below the illustration). From a prime p, like 5 as shown, repeatedly count (shown with arcs) p times multiples of 6, marking out as multiples of p the numbers reached; then on marking out 5p (now 25), repeat from it the same count of px6 for the remaining multiples of p, to be marked out. The same steps when p is 7 are shown in the lower part of the illustration, ending the process.

(5p can likewise be reached by counting; first are counted, mainly backward, the multiples of 6 of which p is a flank, like 1x6 for 5, and then are counted backward from previously marked out 7p, now 35, 2 of those multiples flanked by p, to mark out the opposite flank reached, now 25 again. It should be kept in mind that p is mostly a flank of 2 and more multiples of 6; for instance 11 is a flank of 2x6 = 12, and therefore the count from 7x11 = 77 to 5x11 = 55 is 2x2 multiples of 6.)

There is no need to count the next prime here, 11, since its multiples, as in fact is true of multiples of any prime in the process, are already discounted up to its square, 11 x 11; multiples of the prime by numbers smaller than 11 were considered before when those numbers were multiplied 11 times. In the present case, it is easily seen that the square of 11 is not in the list, which is completed with the square of 10, namely 100. One can usually similarly estimate the square of other upcoming primes, if one does not want to calculate it precisely (this, by the way, also applies to the old sieve).

The images may not be of the quality of printed versions, though I hope the text is clear enough. The four ads published were in succession the first three in the right column and the first in the left column (I elaborated on the text a bit in the first one, immediately to the left).

Planned were the last ads in the right and left column. The story on the "liar" paradox and Gödel is absent in other pages here, although dealt with in my book, if I be permitted to mention it again.

It turns out that I keep adding material, and I might as well when it is valuable to me. Recently I was fortunate to have included a page each about me on two very reputable websites.

The first website is on mathematics, furnishing extensive information in the field. My page describes an angle trisection, which has been a problem throughout history if to be done with a straightedge and compass only. The page is at http://www.cut-the-knot.org/Curriculum/Geometry/Vjecsner.shtml.

The second website is an internationally known one on graphic arts, the people concerned having chanced upon this, my own, website, finding it of sufficient interest for an article on me. The link is http://chapters.aiga.org/content.cfm/his-second-life-an-interview-with-paul-vjecsner.

1 August 2007